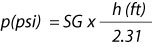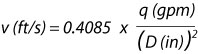Pressure or head

Download this article in pdf format pressure and head as energy

Pressure is a form of energy; head is the height of liquid that produces pressure at the bottom of a containment vessel. The relationship between this height and pressure is:

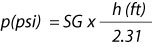
How is pressure a form of energy? If you squeeze an object it will require a force to increase the pressure, the more force you apply the higher the pressure and the more energy required. Try this with a balloon full of air.

It's harder to do that with a liquid but one way is increasing the liquid level in a tank, as it goes up or down the pressure at the bottom increases or decreases, the static head increases or decreases. So where did this energy come from? To increase the pressure at the bottom we used energy from some other device to raise the level of the tank, once again no free lunch.

Energy is this ability to do work that we can store and release later in some fashion. Everything we do in pump systems is a way of storing and transferring energy.

Flow schematics as shown below are a snapshot in time.

We have energy being supplied to the system in the form of the suction static head, we then use the pump's energy to raise and move the liquid, the pump's energy must overcome the friction loss and raise the liquid to a height called the discharge static head or:

H_S = H_T + H_f + H_D

The pump total head is:

H_T = H_f + H_D - H_S

What about the flow rate in all of this? After all we could transfer the liquid at 1 gpm or 1000 gpm. The flow rate is important when it comes to calculating the friction loss, for a given pipe diameter, flow rate provides the velocity

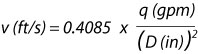
knowing the pipe diameter and the length we can then calculate the fiction loss in terms of head.

What happens to a pump system that has no flow? This can be achieved in many ways, for example we can stop the flow from coming in or out of the system or we can raise the end of the discharge pipe until the flow stops.

How does the pump react to that? The pump's ability to produce head or energy depends on the flow rate that is achieved and the flow rate depends on the characteristics of the system (i.e. pipe length, diameter, elevations, required flow rate).

The particulars of the system (i.e. total static head and friction) will provide us with the total head and knowing the flow will allow us the select a pump. Let's say our calculation indicate that we need a total head of 73 feet at a flow rate of 40 gpm. Because of the large quantity of pumps available on the market, we will find a pump whose capacity will meet those requirements. In figure 3 we see a pump that has a performance curve that meets those requirements.

Pump performance curves have this typical appearance, the total head is highest at zero flow, this head is called the shut-off head, and it gradually decreases as flow is increased. How does the pump know where to operate on its curve, it knows nothing of course it's a piece of steel with a rotating impeller.

When you select a pump for a given system and application the pump has a given size, it might have a 2" diameter suction and a 1" discharge for example. It may come with a motor a 2 hp motor. This pump has the capacity to operate a system at 40 gpm and 70 ft of head, just like a formula 1 car has the engine power and design to go at 200 mph on the straightaway on the racetrack.

Now we can answer the question of what happens when we shut the flow off in a pump system. The pump discharge pressure will increase, meaning the total head will increase and therefore operate at a different place on the performance curve.

This shows that energy or head is not sufficient to produce flow. In Figure 3, the system has zero flow, it can't pump any higher because it has insufficient energy or head.

Therefore it's possible to have the pump produce energy or head without flow. To produce flow we need power. The definition of power is energy per unit time E/t, head is energy per unit weight or h = E/W, therefore power P is:

P = E/t = H x W / t

W/t is the rate of transfer of the weight of the volume of liquid, instead of transferring weight we like to talk about transferring volume, it's more convenient and since liquids are incompressible the volume will not change under different pressures. The volumetric transfer is q in this case in gallons per minute or gpm. Therefore power is:

Since we are now dealing with volumes we need the specific gravity or density of the liquid to convert weight to volume and the constant 3960 to make the units compatible, oh and one last thing since the pump is not 100% efficient so we need to take that into account using the pump efficiency ε

Let's do an example, if our head is 72 ft, the flow rate 40 gpm and say the pump efficiency is 85% which is typical, the result is:

Moving a liquid does appear to be a different proposition than moving a solid. You can hold a solid and move it yourself; you can't do that with liquids. Liquids have to be contained in pipes or vessels and you need pressure to affect them. But the same principles apply.

Let's define a small element of liquid at one end of a horizontal tube; the pressure on one side of the element will be greater on one side, which will make it move forward. As this element moves another element behind replaces it also moving forward. The complete volume of the liquid is represented by small elements moving forward and being acted on by pressure. The pressure decreases and eventually reaches zero as it gets to the end of the tube, this is because as the element gets closer to the open end there is less and less friction because the tube is getting shorter therefore less pressure is required to drive the element forward.

We can expand our small element, since one end of the element is open to atmosphere the pressure must be zero therefore p₁ = 0, the force corresponding to p₂ is F₂ = p₂ x A. Energy is defined as a force times a distance E = F x d, therefore the energy of friction is E_f = F₂ x d and then E_f = p₂ x A x d, p₂ is the pressure required to overcome friction therefore p₂ = p_f or

E_f = p₂ x d = p_f x d

We can convert pressure to head

p (psi) =SG x h (feet) / 2.31

Therefore

E_f = SG x H_f / 2.31 x A x d

SG x A x D /2.31 is equal to weight, therefore H_f is energy per unit weight as expected.

H_f is the friction head which we can find form tables or other methods.

This is another reason why head is such a useful concept because it is energy per unit weight and we are moving small units of weight steadily forward.